The relationship between the ratios of a right-angled triangle’s sides and angles is the subject of trigonometry. To analyses, this relationship, trigonometric ratios like sine, cosine, tangent, cotangent, secant, and cosecant are used. The concept of trigonometry was developed by the Greek mathematician Hipparchus, while the term trigonometry is a 16th-century Latin derivative.
Simple Trigonometry
In the information that follows, we’ll look at the theoretical foundations of trigonometry, along with its many identities, formulas, and applications.
The three basic trigonometric operations are sine, cosine, and tangent. The cotangent, secant, and cosecant functions are built on these three fundamental functions.
These functions serve as the foundation for all trigonometry concepts. Therefore, we must first learn these functions in order to fully comprehend trigonometry.
Who will help me complete my online course now that I feel comfortable? Since it allows for the use of both students and teachers as well as cutting-edge study techniques that aid students in understanding the subject material, online education provides many benefits for both parties.
If the angle of the right-angled triangle is, then
Hypotenuse/perpendicular = Sin
Hypotenuse/Base = Cos
Tan is the base or perpendicular.
The hypotenuse is the side that faces the right angle.
The foundations of trigonometry cover angles’ measurement and problems involving them.
Trigonometry’s three fundamental operations are sine, cosine, and tangent.
All of the fundamental trigonometry principles are constructed using these functions.
As a result, in order to understand trigonometry, we first strive to grasp the understanding of functions and their associated formulas.
Equations of trigonometric functions that hold under all conditions are known as trigonometry identities. Trigonometry identities are frequently used to comprehend fundamental mathematical concepts and resolve other mathematical difficulties in addition to solving trigonometry problems.
Trigonometry of angles
In trigonometry problems, the trigonometric angles 0°, 30°, 45°, 60°, and 90° are frequently used. Certain trigonometric ratios, including sine, cosine, and tangent, are simple to remember. We will also display the table that lists the values and ratios for each angle. To obtain these angles, we must first draw a right-angled triangle with one sharp angle equal to the trigonometric angle. Regarding these angles, the corresponding ratio will be discussed.
Using a triangle with a right angle as an illustration
- Perpendicular/hypotenuse = Sin
- or sin(P/H) = 1
Similarly,
- (Base/Hypotenuse) = cos-1
- (Perpendicular/Base) = tan-1
An explanation of the Delta Symbol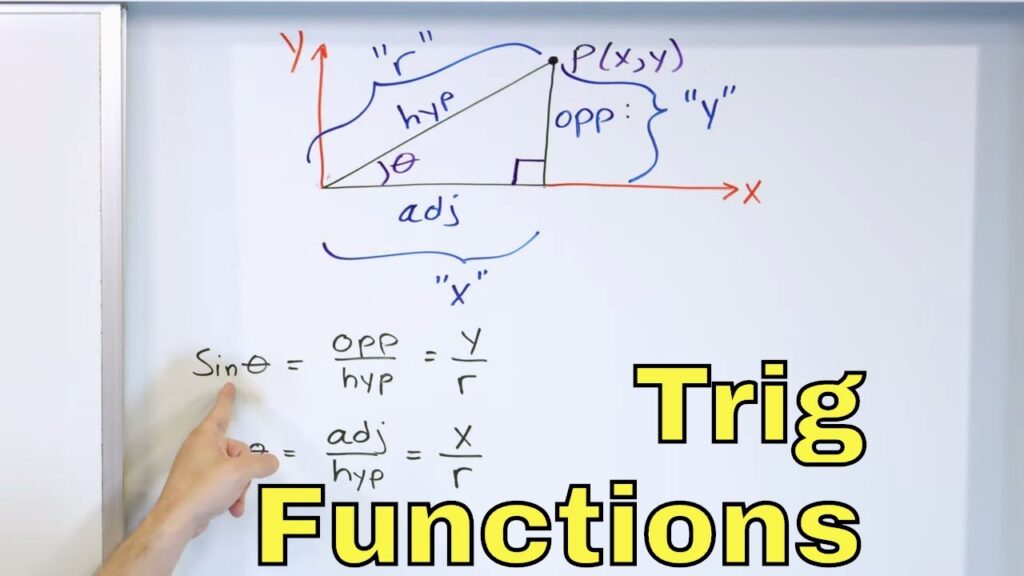
The fourth letter in the Greek alphabet is called the delta. The Phoenician letter dalet served as the foundation for the delta. The delta is a reasonably common symbol in mathematics.
The mathematical symbol for a number, function, set, or equation is called a delta. Here, students can read more about the mathematical importance of the delta symbol.
Change or “the change” is typically represented in mathematics by an uppercase delta (). Consider a scenario in which the variable x symbolizes an object’s motion. “X” denotes “the change in movement” as a result. Researchers in a range of scientific fields employ this mathematical interpretation of delta.
In the language of geometry, an angle can be expressed as a lowercase delta () in any geometric shape.
This is mainly because geometry derives from Euclid’s classical Greek writings. Mathematicians consequently utilized Greek letters to represent their angles.
It is not required to understand or be familiar with the Greek alphabet. Since the letters are merely symbols for angles, this is the case.
The derivative of a function takes into account even the slightest changes to one of its variables. There are situations when a derivative is represented by the Roman letter “d.”
Partial derivatives are distinct from normal derivatives. Even though the function has many versions, only one variable is taken into account in this case. The other components are still present, without a doubt.
A Capital D (Kronecker Delta)
In more complex mathematics, lowercase delta () has a considerably more specialised application. Additionally, the calculus symbol for a change in a variable’s value is lowercase delta.
As an example, consider the Kronecker delta case. The Kronecker delta shows a link between two integral variables. If the two variables are equal, this value is 1. Additionally, if the two variables are not the same, this equals 0.
Making use of trigonometry
Trigonometry has historically been used in fields including surveying, astronomy, and architecture. Some of its applications are
Included are a wide range of disciplines, such as physical sciences, astronomy, acoustics, navigation, electronics, oceanography, and seismology.
It is possible to calculate the length of long rivers, the height of a mountain, etc.
Astronomers have calculated the positions of the sun, moon, and stars using spherical trigonometry.
Calculating height and distance is one of the most crucial applications for trigonometry in daily life. The principles of trigonometry are frequently applied in a variety of disciplines, such as criminology, marine biology, aviation, and navigation.
A methodical application of matter is mathematics. It is presumable that the subject causes a man to become methodical or systematic. Strong reasoning, inventiveness, abstract or spatial thinking, critical thinking, problem-solving skills, and even exceptional communication skills are some of the attributes that mathematics fosters.
Numerous occupations, such as accounting, finance, banking, engineering, and software, as well as everyday tasks like keeping time and driving heavily rely on mathematics. Strong mathematical skills are necessary for these positions, and mathematicians are needed by scientists conducting scientific experiments. They serve as a language for expressing the endeavors and achievements of scientists.
You can obtain college credit for your math courses. Along with gaining a thorough comprehension of each mathematical concept, you can also improve your analytical skills.
Conclusion:
Concepts concerning the outer world can be represented by combining mathematical symbols. Occasionally, these symbols can represent numbers or take on a more abstract meaning, denoting regions, symmetries, or groups. These symbols paired with mathematical operations like addition, subtraction, or multiplication, to mention a few, result in mathematical expressions.






Discussion about this post